Blistering is a mechanism by which a delaminated film can release compressive stresses. This happens for example upon cooling films deposited at high temperature on a substrate with a larger thermal expansion coefficient. The same phenomenon can be observed compressing paper sheets partially glued to a substrate (see Gioia, DeSimone, Ortiz, Cuitino, preprint cond-mat/0005058).

Observed shapes are reminiscent of sand heaps, and the height is in many cases roughly proportional to the distance function to the boundary. The distance function is, in turn, the maximal pointwise solution to the eikonal equation which vanishes at the boundary.

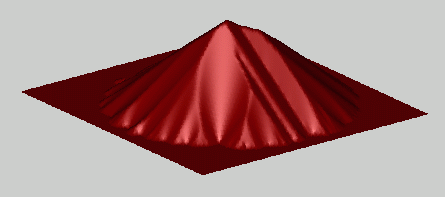
A sand heap: side and top views (you can generate these shapes with the matlab script given in Alouges and DeSimone, J. of Elasticity 55, 231-237, 1999)

A natural approach to study this phenomenon quantitatively in the limit of small film thickness and in a geometrically linear setting is to use the Föppl-von Kármán theory of plates. This was done by Ortiz and Gioia [J. Mech. Phys. Solids 42, 531 (1994)]. They showed that, if the in-plane displacement u is neglected, then the leading-order term in the energy is minimized by solutions of the eikonal equation. It has been later shown that, in fact, in the limit of small film thickness energy minimizers with u=0 do satisfy the eikonal equation [DeSimone, Kohn, Müller, Otto, Preprint 23/1999 and Ambrosio, DeLellis and Mantegazza, Calc. Var. 9, 327 (2000)].

The natural next step was to remove the assumption u=0, which leads to a scaling of the energy linear in h, as the film thickness h tends to zero. Jin and Sternberg (Proc. Roy. Soc. Edin, to appear) have considered a larger (but still restricted) class of candidate minimizers (with nonzero u), and obtained a faster decay, with exponent 5/3. The correct exponent, considering the full class of candidate minimizers, is 2. This result was obtained by Ben Belgacem, Conti, DeSimone and Müller, J. Nonlinear Sci. 10, 661-683 (2000) and, independently, by Jin and Sternberg (J. Math. Phys., to appear).

			Oscillations and branching near a straight boundary

Our result relies on a construction where the in-plane component u has gradient of order 1 and branching develops near the boundary (see the Figure above). This is a necessary condition to achieve the optimal energy scaling. Hence, if u is indeed small, as Ortiz and Gioia suggested, the system must develop fine-scale oscillations, at least in the in-plane component.

Forthcoming work from our group shows that this scenario holds also in the geometrically nonlinear regime.