Number Theory and Quantum Mechanics
“What’s one and one and one and and one and one and one and one and one and one and one?”
“I don’t know”, said Alice, “I lost count”.
“She can’t do Addition”, the Red Queen interrupted.
-- Lewis Carroll, Through the Looking Glass.

Number theory has always been an endless source of delights for the simplest formulation of its problems and the deepest reasoning paths of their solutions.
Charming examples are drawn from elementary number theory, which deals with divisibility and prime factorization, residue classes, congruences, diophantine equations, continued fraction approximations and sieves. Others comes from combinatorial number theory and the sophisticate branch of elliptic curves and modular forms - a field full of amazing Ramanujan’s identities which enter the solution of statistical models as the 8-vertex model,
and also vital to the solution of various Diophantine problems, such as Fermat’s Last Theorem.
Elegant applications in physics of this kind of discrete mathematics are, among others (in random order) :
(a) the ADE classification of the modularinvariant partition functions of Conformal Field Theory; (b) the generating functions associated to the characters of the Virasoro algebra; (c) the remarkable structure of quasi-crystals, which share important properties with both perfect crystals and amorphus substances; (d) the appearance of Fibonacci numbers in non-abelian anyons; (e) the Hofstadter butterfly, i.e. the self-similar structure of the allowed energy levels of an electron in a crystal lattice subject to a magnetic field.

Hofstadter’s butterfly
Particularly interesting is the interplay between number theory and quantum mechanics: the discrete pattern of Quantum Mechanics emerges, in particular, in the structure of the energy levels. A well-known problem is the Riemann’s conjecture about the zeros of the Riemann zeta function and the possibility to regard their imaginary part as eigenvalues of a quantum hamiltonian. Michael Berry pointed out that the statistical distribution of these zeros has shown a remarkable connection with the random matrix theory and the theory of chaotic systems. Progress on this problem has been recently achieved by German Sierra in a series of paper. As shown by Bernard Julia, the Riemann zeta function can also have an interesting interpretation in quantum statistical mechanics.
Dual to the Riemann zeros there are the prime numbers, the building blocks of number theory. No one has ever found a formuta for n-th prime, nor a formula for the prime that immediately follows a given one. In fact, no one has ever found a simple rule for finding any prime larger than a given one, and all such questions appear to be hopelessly out of reach. There is just no apparent reason why one number is prime while another is not.
Number-town and its prime number boulevards
But whatever eccentricities the primes may exhibit “on the small scale”, their aggregate behavior is orderely and predictable. The function which counts the primes becomes more and more smooth on a large scale and can be exactly determined. Fascinating patters emerge as well when the natural numbers are plotted in spiral lattices, where it happens that many primes fall in straight lines: this pattern of straight lines shows that there exists many quadratic polynomial functions which produce large sequences of primes.
To decide whether a natural number is a prime, and in case of negative answer, what is its prime number decomposition are important mathematical questions, that can also have relevant implications for implementing public-key encryption and deciphering of secret messages.

Leaving behind any pretension of rigorousness, in the paper “Quantum Mechanical Potential for the Prime Numbers” by the author it was proposed to use methods of semi-classical quantum mechanics for identify a one-dimensional potential whose eigenvalues match the prime numbers. If this initial ansatz could be further refined and made exact, and if such a potential can be realized in the laboratory, this implies that the primality test and the prime number decomposition of a composite natural number can be solved by the sole use of physical laws: indeed it would be sufficient to rely on scattering experiments.
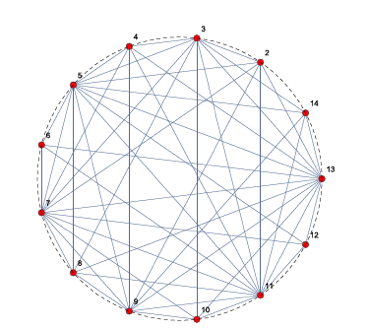
In the paper “Coprime Quantum Chain” it was introduced and studied an amazing quantum chain whose distinctive interaction is based on the coprimality matrix of the next-neighbor occupation numbers (assumed to be integers): for the ferromagnetic case, this matrix assigns lower energy to configurations where n.n. occupation numbers share a common divisor, while for the anti-ferromagnetic case it assigns lower energy to configurations where the two consecutive integers are coprime. The
coprime chain, both in the ferro and anti-ferromagnetic cases, may present an exponential number of ground states whose values can be exactly computed by means of graph theoretical tools. In the ferromagnetic case there are generally also frustration phenomena. A fine tuning of local operators may lift the exponential ground state degeneracy and, according to which operators are switched on, the system may be driven into different classes of universality, among which the Ising or Potts universality class. In the paper where all properties of this amusing model are discussed there is also a very interesting appendix written by Don Zagier on the exact eigenvalues and eigenvectors of the coprimality matrix in the limit q→∞
Relevant Publications
G. Mussardo, The Quantum Mechanical Potential for the Prime Numbers, arXiv:cond-mat/9712010
G. Mussardo, G. Giudici, J. Viti, The Coprime Quantum Chain, J.Stat.Mech. 1703 (2017) no.3, 033104, https://arxiv.org/abs/1701.02317
Main References
M.R. Scroeder, Number Theory in Science and Communication, Springer-Verlag, 1990.
R.M. Young, Excursions in Calculus. An Interplay of the Continuous and the Discrete, Dolciani Mathematical Expositions Number 13.
• G.E. Andrews, Number Theory, Dover Publ. Inc, New York 1971.
• A. Weil, Number Theory, an Approach Through History: from Hammurabi to Legendre, Birkhauser, Berlin 1983.
H.M. Edwards, Riemann function, Academic Press, 1964.
Frontiers in Number Theory, Physics, and Geometry I On Random Matrices, Zeta Functions, and Dynamical Systems, Eds: P. Cartier, B. Julia, P. Moussa, P. Vanhove. Springer Verlag, Berlin, 2006.
M.V. Berry, in Quantum Chaos and Statistical Nuclear Physics. Eds. T.H. Seligman and H. Nishioka, Lecture Notes in Physics, No. 263, Springer Verlag, New York, 1986.
A. Connes, Trace formula in noncommutative geometry and the zeros of the Riemann zeta function, Selecta Mathematica (New Series) 5 (1999) 29.
M.V. Berry and J.P. Keating, H=xp and the Riemann zeros, in Supersymmetry and Trace Formulae: Chaos and Disorder, ed. J.P. Keating, D.E. Khmelnitskii and I. V. Lerner, Kluwer 1999.
G. Sierra, P.K. Towsend, Landau levels and Riemann zeros, Phys. Rev. Lett. 101; 110201, 2008,
G. Sierra, A quantum mechanical model of the Riemann zeros, New J. Phys. 10: 022016, 2008,